Integers in Euclidean Space Order

Posted on by

Consider the factored integers N=[(0), (1), (2), (3), (2,2), 5, (2,3), 7, (2,2,2)...], with each factor representing a point in Euclidean space. Each of these points is at a distance d from the origin, and can be ordered by d.

-- Ieso.hs
-- Integers in Euclidean space order

-- Uses the Haskell Number Theory functions, from the "Haskell for Maths"
-- site: http://www.polyomino.f2s.com/david/haskell/numbertheory.html
import Data.List
import Primes

-- Return distance between two points in Euclidean space. Points are lists 
-- of possibly unequal lengths, padded with zeros as needed.
dist_points x y = sqrt (realToFrac ((foldl (+) 0 (map sq (zipWith (-) x1 y1)))))
           where x1 = pad (lengthLongest [x,y]) x
                 y1 = pad (lengthLongest [x,y]) y
                 sq x = x * x
                 lengthLongest l = maximum (map length l)
                 pad len x = (x ++ zeroPadding) 
                     where zeroPadding = genericTake (len - genericLength x) (repeat 0)

-- Return list of all prime factors of n
primeFactors 1 = [1]
primeFactors n = flatten (map expandedFactors (primePowerFactors n))
            where
              expandedFactors x = genericTake (snd x) (repeat (fst x))
              flatten :: [[a]] -> [a]
              flatten = foldl (++) []

-- Return distance between two factored integers 
distance n m = dist_points (primeFactors n) (primeFactors m)

-- Return list of distances between origin and integers up to n,
-- sorted by distance d. Where ties occur, the lowest n comes first, i.e., 
-- where d=5 for both n=48 and n=5, the order is ... 5, 48 ....
-- Neil Sloane has suggested a different way of breaking ties is use of 
-- lexicographic order, ie for n=48 [2,2,2,3] comes before n=5 [5] - see
-- below.
distanceOrigin n = map snd (sort d)
                   where
                     d = [(distance 0 i, i) | i <- [0..n]]

-- In order to list the integers within a certain distance d from the origin,
-- we have to calculate distances up to 2^(d^2/4), e.g. to list ALL 
-- integers within a distance of 10, use range 2...2^25.

-- I believe a list is "complete" up to where a power of 2 appears, 
-- because numbers greater than that power of 2 can't be closer 
-- to the origin (except ties, depending on they are handled). However, 
-- I am curious about how to determine the  minimum number of 
-- integers you have to calculate in order to make a list complete, 
-- e.g., how high do we have to look to be sure we've found the
-- 20 integers closest to the origin? 

main = do
  -- Calculate integers within ~7 units of origin
  print "n ordering for ties"
  let d = [(distance 0 i, i) | i <- [0..8192]]
  print (map snd (sort d))

  -- Recalculate using lexicographic order to break ties
  print "lexicographical ordering for ties"  
  let l = [(distance 0 i, primeFactors i, i) | i <- [0..8192]]
  print (map lastx (sort l))
        where
          lastx (x, y, z) = z

For example, d=8.66 for N=125 at the point (5,5,5), while d=5.29 for N=128 at (2,2,2,2,2,2,2) :

*Ieso> distance 0 125
8.660254037844387
*Ieso> distance 0 128
5.291502622129181

Here is a simple view of several of these integers that lie in 3-space, with the distance between the origin and N=125 highlighted.
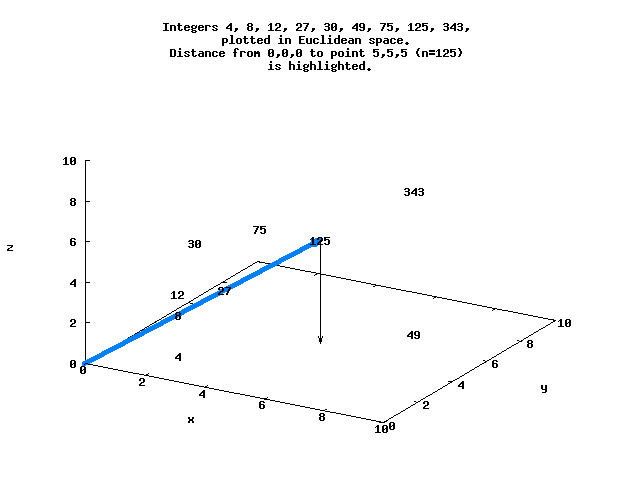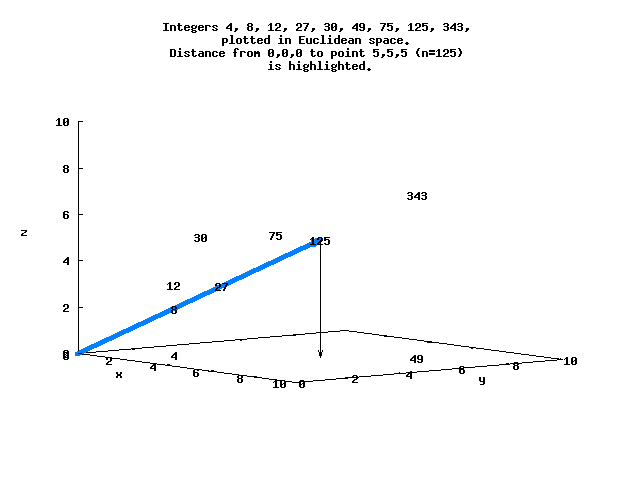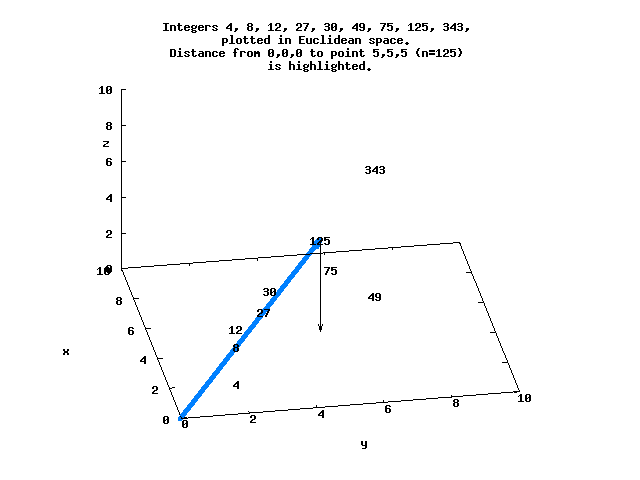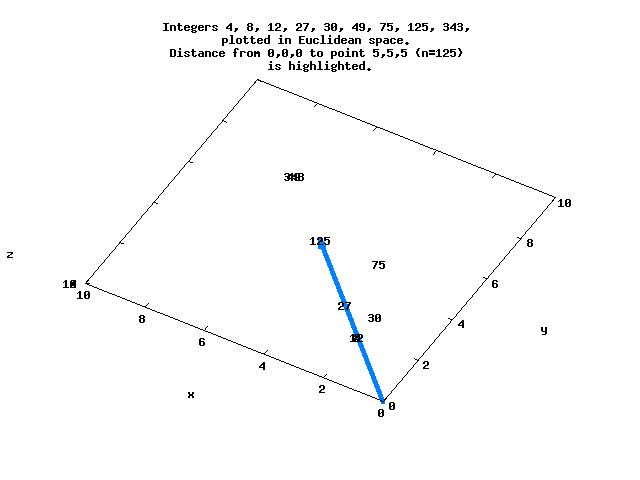
3d.gif
The sequence N=[0..8192] in Euclidean space ordered by d begins

 
*Ieso> distanceOrigin 8192
0,1,2,4,3,8,6,16,12,9,32,24,18,64,5,48,36,27,128,10,96,72,54,256,20,192,15,144,108,81,512,40,384,30,288,216,162,1024

(One way to think about this may be that integers with higher Ω(n) should be relatively clustered around the origin).

(The sequence is listed as OEIS A168521).

Comments are closed.